gerut.blogg.se - A negative simple linear regression equation slope

Note: We used the Inverse t Distribution Calculator to find the t critical value that corresponds to a 95% confidence level with 13 degrees of freedom.Face validity and content validity are similar in that they both evaluate how suitable the content of a test is. Since this confidence interval doesn’t contain the value 0, we can conclude that there is a statistically significant association between hours studied and exam score. The 95% confidence interval for the regression slope is. We can use the following formula to calculate a 95% confidence interval for the slope:

This tells us that each additional one hour increase in studying is associated with an average increase of 1.982 in exam score. The value for the regression slope is 1.982. Using the coefficient estimates in the output, we can write the fitted simple linear regression model as: We can perform simple linear regression in Excel and receive the following output: Suppose we’d like to fit a simple linear regression model using hours studied as a predictor variable and exam score as a response variable for 15 students in a particular class: Example: Confidence Interval for Regression Slope The following example shows how to calculate a confidence interval for a regression slope in practice.

se(b 1) = The standard error of b 1 shown in the regression table.

t 1-∝/2, n-2 = The t critical value for confidence level 1-∝ with n-2 degrees of freedom where n is the total number of observations in our dataset.

b 1 = Slope coefficient shown in the regression table.

We can use the following formula to calculate a confidence interval for the value of β 1, the value of the slope for the overall population:Ĭonfidence Interval for β 1: b 1 ± t 1-α/2, n-2 * se(b 1) Often we’re interested in the value for b 1, which tells us the average change in the response variable associated with a one unit increase in the predictor variable.

b 0: The intercept of the regression line.

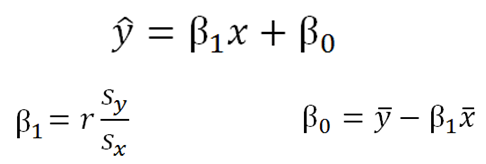
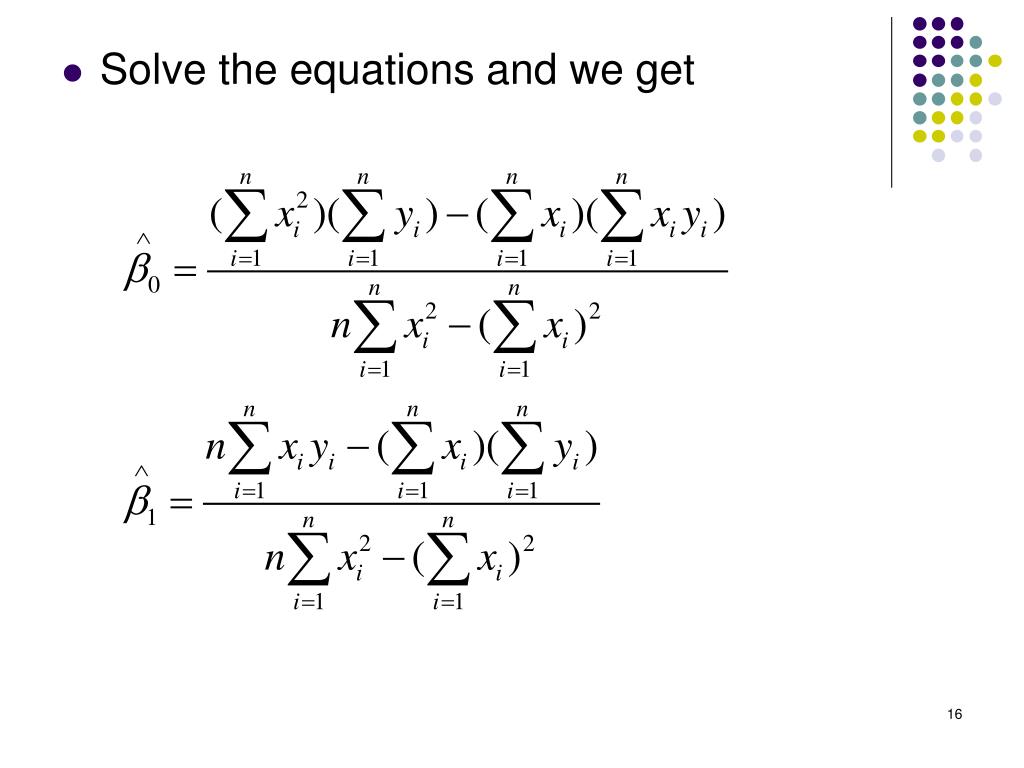
This method finds a line that best “fits” a dataset and takes on the following form: Simple linear regression is used to quantify the relationship between a predictor variable and a response variable.